[SGK Toán Lớp 10 Kết nối tri thức] Bài 11. Tích vô hướng của hai vecto
Hướng dẫn học bài: Bài 11. Tích vô hướng của hai vecto - Môn Toán học Lớp 10 Lớp 10. Đây là sách giáo khoa nằm trong bộ sách 'SGK Toán Lớp 10 Kết nối tri thức Lớp 10' được biên soạn theo chương trình đổi mới của Bộ giáo dục. Hi vọng, với cách hướng dẫn cụ thể và giải chi tiết các bé sẽ nắm bài học tốt hơn.
Đề bài
Cho tam giác ABC có trọng tâm G. Chứng minh rằng với mọi điểm M, ta có:
\(M{A^2} + M{B^2} + M{C^2} = 3M{G^2} + G{A^2} + G{B^2} + G{C^2}\)
Phương pháp giải - Xem chi tiết
+) \(M{A^2} = {\overrightarrow {MA} ^2}\)
+) Với 3 điểm M, A, G bất kì ta có: \(\overrightarrow {MG} + \overrightarrow {GA} = \overrightarrow {MA} \)
+) G là trọng tâm tam giác ABC thì: \(\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} = \overrightarrow 0 \)
Lời giải chi tiết
Ta có:
\(\begin{array}{l}M{A^2} + M{B^2} + M{C^2} = {\overrightarrow {MA} ^2} + {\overrightarrow {MB} ^2} + {\overrightarrow {MC} ^2}\\ = {\left( {\overrightarrow {MG} + \overrightarrow {GA} } \right)^2} + {\left( {\overrightarrow {MG} + \overrightarrow {GB} } \right)^2} + {\left( {\overrightarrow {MG} + \overrightarrow {GC} } \right)^2}\\ = {\overrightarrow {MG} ^2} + 2\overrightarrow {MG} .\overrightarrow {GA} + {\overrightarrow {GA} ^2} + {\overrightarrow {MG} ^2} + 2\overrightarrow {MG} .\overrightarrow {GB} + {\overrightarrow {GB} ^2} + {\overrightarrow {MG} ^2} + 2\overrightarrow {MG} .\overrightarrow {GC} + {\overrightarrow {GC} ^2}\\ = 3{\overrightarrow {MG} ^2} + 2\overrightarrow {MG} .\left( {\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} } \right) + {\overrightarrow {GA} ^2} + {\overrightarrow {GB} ^2} + {\overrightarrow {GC} ^2}\\ = 3{\overrightarrow {MG} ^2} + 2\overrightarrow {MG} .\overrightarrow 0 + {\overrightarrow {GA} ^2} + {\overrightarrow {GB} ^2} + {\overrightarrow {GC} ^2}\end{array}\)
( do G là trọng tâm tam giác ABC)
\(\begin{array}{l} = 3{\overrightarrow {MG} ^2} + {\overrightarrow {GA} ^2} + {\overrightarrow {GB} ^2} + {\overrightarrow {GC} ^2}\\ = 3M{G^2} + G{A^2} + G{B^2} + G{C^2}\end{array}\) (đpcm).
Đề bài
Chứng minh rằng với mọi tam giác ABC, ta có:
\({S_{ABC}} = \frac{1}{2}\sqrt {{{\overrightarrow {AB} }^2}.{{\overrightarrow {AC} }^2} - {{\left( {\overrightarrow {AB} .\overrightarrow {AC} } \right)}^2}} .\)
Phương pháp giải - Xem chi tiết
Biến đổi vế trái, đưa về công thức \({S_{ABC}} = \dfrac{1}{2}bc.\sin A\)
+) \(\overrightarrow {AB} .\overrightarrow {AC} = AB.AC.\cos \left( {\overrightarrow {AB} ,\overrightarrow {AC} } \right)\)
+) \({\sin ^2}\alpha = 1 - {\cos ^2}\alpha \) với mọi \(\alpha \).
Lời giải chi tiết
Đặt \(A = \dfrac{1}{2}\sqrt {{{\overrightarrow {AB} }^2}.{{\overrightarrow {AC} }^2} - {{\left( {\overrightarrow {AB} .\overrightarrow {AC} } \right)}^2}} \)
\(= \dfrac{1}{2}\sqrt { A{B^2}.A{C^2}- {{\left(|{\overrightarrow {AB}| .|\overrightarrow {AC}|. \cos BAC} \right)}^2}} \)
\(\begin{array}{l} \Rightarrow A = \dfrac{1}{2}\sqrt {A{B^2}.A{C^2} - {{\left( {AB.AC.\cos A} \right)}^2}} \\ \Leftrightarrow A = \dfrac{1}{2}\sqrt {A{B^2}.A{C^2} - A{B^2}.A{C^2}.{{\cos }^2}A }\\ \Leftrightarrow A = \dfrac{1}{2}\sqrt {A{B^2}.A{C^2}\left( {1 - {{\cos }^2}A} \right)} \end{array}\)
Mà \(1 - {\cos ^2}A = {\sin ^2}A\)
\( \Rightarrow A = \dfrac{1}{2}\sqrt {A{B^2}.A{C^2}.{{\sin }^2}A} \)
\( \Leftrightarrow A = \dfrac{1}{2}.AB.AC.\sin A\) (Vì \({0^o} < \widehat A < {180^o}\) nên \(\sin A > 0\))
Do đó \(A = {S_{ABC}}\) hay \({S_{ABC}} = \dfrac{1}{2}\sqrt {{{\overrightarrow {AB} }^2}.{{\overrightarrow {AC} }^2} - {{\left( {\overrightarrow {AB} .\overrightarrow {AC} } \right)}^2}} .\) (đpcm)
Đề bài
Trong mặt phẳng tọa độ Oxy, cho ba điểm không thẳng hàng A (-4; 1), B (2;4), C (2; -2)
a) Giải tam giác
b) Tìm tọa độ trực tâm H của tam giác ABC.
Phương pháp giải - Xem chi tiết
a) Độ dài vectơ \(\overrightarrow {AB} (x;y)\) là \(\left| {\overrightarrow {AB} } \right| = \sqrt {{x^2} + {y^2}} \)
b) Chỉ ra \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \) và \(\overrightarrow {BH} .\overrightarrow {CA} = \overrightarrow 0 \) từ đó tìm tọa độ của H.
Lời giải chi tiết
a) Ta có:
\(\left\{ \begin{array}{l}\overrightarrow {AB} = (2 - ( - 4);4 - 1) = (6;3)\\\overrightarrow {BC} = (2 - 2; - 2 - 4) = (0; - 6)\\\overrightarrow {AC} = (2 - ( - 4); - 2 - 1) = (6; - 3)\end{array} \right.\)\( \Rightarrow \left\{ \begin{array}{l}AB = \left| {\overrightarrow {AB} } \right| = \sqrt {{6^2} + {3^2}} = 3\sqrt 5 \\BC = \left| {\overrightarrow {BC} } \right| = \sqrt {{0^2} + {{( - 6)}^2}} = 6\\AC = \left| {\overrightarrow {CA} } \right| = \sqrt {{6^2} + {{( - 3)}^2}} = 3\sqrt 5 .\end{array} \right.\)
Áp dụng định lí cosin cho tam giác ABC, ta có:
\(\cos \widehat A = \frac{{{b^2} + {c^2} - {a^2}}}{{2bc}} = \frac{{{{\left( {3\sqrt 5 } \right)}^2} + {{\left( {3\sqrt 5 } \right)}^2} - {{\left( 6 \right)}^2}}}{{2.3\sqrt 5 .3\sqrt 5 }} = \frac{3}{5}\)\( \Rightarrow \widehat A \approx 53,{13^o}\)
\(\cos \widehat B = \frac{{{a^2} + {c^2} - {b^2}}}{{2ac}} = \frac{{{{\left( 6 \right)}^2} + {{\left( {3\sqrt 5 } \right)}^2} - {{\left( {3\sqrt 5 } \right)}^2}}}{{2.6.3\sqrt 5 }} = \frac{{\sqrt 5 }}{5}\)\( \Rightarrow \widehat B \approx 63,{435^o}\)
\( \Rightarrow \widehat C \approx 63,{435^o}\)
Vậy tam giác ABC có: \(a = 6;b = 3\sqrt 5 ;c = 3\sqrt 5 \); \(\widehat A \approx 53,{13^o};\widehat B = \widehat C \approx 63,{435^o}.\)
b)
Gọi H có tọa độ (x; y)
\( \Rightarrow \left\{ \begin{array}{l}\overrightarrow {AH} = (x - ( - 4);y - 1) = (x + 4;y - 1)\\\overrightarrow {BH} = (x - 2;y - 4)\end{array} \right.\)
Lại có: H là trực tâm tam giác ABC
\( \Rightarrow AH \bot BC\) và \(BH \bot AC\)
\( \Rightarrow \left( {\overrightarrow {AH} ,\overrightarrow {BC} } \right) = {90^o} \Leftrightarrow \cos \left( {\overrightarrow {AH} ,\overrightarrow {BC} } \right) = 0\) và \(\left( {\overrightarrow {BH} ,\overrightarrow {AC} } \right) = {90^o} \Leftrightarrow \cos \left( {\overrightarrow {BH} ,\overrightarrow {AC} } \right) = 0\)
Do đó \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \) và \(\overrightarrow {BH} .\overrightarrow {AC} = \overrightarrow 0 \).
Mà: \(\overrightarrow {BC} = (0; - 6)\)
\( \Rightarrow (x + 4).0 + (y - 1).( - 6) = 0 \Leftrightarrow - 6.(y - 1) = 0 \Leftrightarrow y = 1.\)
Và \(\overrightarrow {AC} = (6; - 3)\)
\(\begin{array}{l} \Rightarrow (x - 2).6 + (y - 4).( - 3) = 0\\ \Leftrightarrow 6x - 12 + ( - 3).( - 3) = 0\\ \Leftrightarrow 6x - 3 = 0\\ \Leftrightarrow x = \frac{1}{2}.\end{array}\)
Vậy H có tọa độ \(\left( {\frac{1}{2}}; 1 \right)\)
Đề bài
Trong mặt phẳng tọa độ Oxy, cho hai điểm A (1; 2), B(-4; 3). Gọi M (t; 0) là một điểm thuộc trục hoành.
a) Tính \(\overrightarrow {AM} .\overrightarrow {BM} \) theo t.
b) Tính t để \(\widehat {AMB} = {90^o}\)
Phương pháp giải - Xem chi tiết
+) Nếu vecto \(\overrightarrow {AM} (x;y)\) và \(\overrightarrow {BM} (a;b)\) thì \(\overrightarrow {AM} .\overrightarrow {BM} = xa + yb\)
+) \(\widehat {AMB} = {90^o} \Leftrightarrow AM \bot BM\)
Lời giải chi tiết
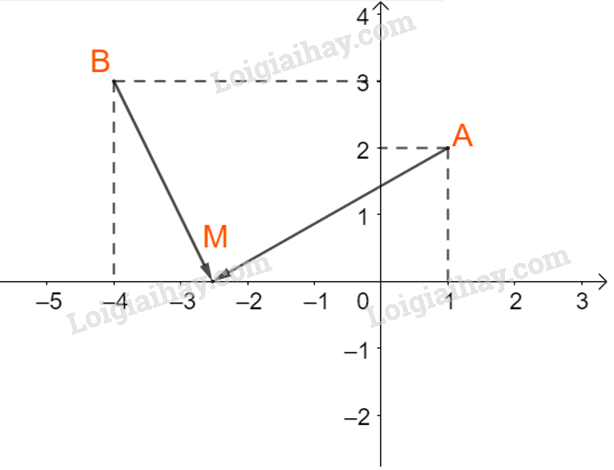
a)
Ta có: A (1; 2), B(-4; 3) và M (t; 0).
\(\begin{array}{l} \Rightarrow \overrightarrow {AM} = (t - 1; - 2),\;\overrightarrow {BM} = (t + 4; - 3)\\ \Rightarrow \overrightarrow {AM} .\overrightarrow {BM} = (t - 1)(t + 4) + ( - 2)( - 3)\\\quad \quad \quad \quad \quad \quad= {t^2} + 3t + 2.\end{array}\)
b)
Để \(\widehat {AMB} = {90^o}\) hay \(AM \bot BM\) thì \(\overrightarrow {AM} .\overrightarrow {BM} = 0\)
\(\begin{array}{l} \Leftrightarrow {t^2} + 3t + 2 = 0\\ \Leftrightarrow \left[ \begin{array}{l}t = - 1\\t = - 2\end{array} \right.\end{array}\)
Vậy t = -1 hoặc t = -2 thì \(\widehat {AMB} = {90^o}\).
Đề bài
Tìm điều kiện của \(\overrightarrow u ,\;\overrightarrow v \) để:
a) \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|\)
b) \(\overrightarrow u .\;\overrightarrow v = - \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|\)
Phương pháp giải - Xem chi tiết
Tích vô hướng \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
Lời giải chi tiết
a)
Ta có: \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \left( {\overrightarrow u ,\;\overrightarrow v } \right) = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|\).
Khi đó \( \cos \left( {\overrightarrow u ,\;\overrightarrow v } \right) = 1\) suy ra \( \left( {\overrightarrow u ,\;\overrightarrow v } \right) = {0^o}\).
Nói cách khác: \(\overrightarrow u ,\;\overrightarrow v \) cùng hướng.
b)
Ta có: \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \left( {\overrightarrow u ,\;\overrightarrow v } \right) =- \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|\).
Khi đó \( \cos \left( {\overrightarrow u ,\;\overrightarrow v } \right) = - 1 \) suy ra \( \left( {\overrightarrow u ,\;\overrightarrow v } \right) = {180^o}\).
Nói cách khác: \(\overrightarrow u ,\;\overrightarrow v \) ngược hướng.
Đề bài
Trong mặt phẳng tọa độ Oxy, hãy tính góc giữa hai vectơ \(\overrightarrow a \) và \(\overrightarrow b \) trong mỗi trường hợp sau:
a) \(\overrightarrow a = ( - 3;1),\;\overrightarrow b = (2;6)\)
b) \(\overrightarrow a = (3;1),\;\overrightarrow b = (2;4)\)
c) \(\overrightarrow a = ( - \sqrt 2 ;1),\;\overrightarrow b = (2; - \sqrt 2 )\)
Phương pháp giải - Xem chi tiết
Tính góc giữa hai vectơ dựa vào tích vô hướng: \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}}\)
Lời giải chi tiết
a)
\(\overrightarrow a .\overrightarrow b = ( - 3).2 + 1.6 = 0\)
\( \Rightarrow \overrightarrow a \bot \overrightarrow b \) hay \(\left( {\overrightarrow a ,\overrightarrow b } \right) = {90^o}\).
b)
\(\left\{ \begin{array}{l}\overrightarrow a .\overrightarrow b = 3.2 + 1.4 = 10\\|\overrightarrow a |\, = \sqrt {{3^2} + {1^2}} = \sqrt {10} ;\;\,|\overrightarrow b |\, = \sqrt {{2^2} + {4^2}} = 2\sqrt 5 \end{array} \right.\)
\(\begin{array}{l} \Rightarrow \cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{10}}{{\sqrt {10} .2\sqrt 5 }} = \frac{{\sqrt 2 }}{2}\\ \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = {45^o}\end{array}\)
c) Dễ thấy: \(\overrightarrow a \) và \(\overrightarrow b \) cùng phương do \(\frac{{ - \sqrt 2 }}{2} = \frac{1}{{ - \sqrt 2 }}\)
Hơn nữa: \(\overrightarrow b = \left( {2; - \sqrt 2 } \right) = - \sqrt 2 .\left( { - \sqrt 2 ;1} \right) = - \sqrt 2 .\overrightarrow a \;\); \( - \sqrt 2 < 0\)
Do đó: \(\overrightarrow a \) và \(\overrightarrow b \) ngược hướng.
\( \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = {180^o}\)
Chú ý:
Khi tính góc, ta kiểm tra các trường hợp dưới đây trước:
+ \(\left( {\overrightarrow a ,\overrightarrow b } \right) = {90^o}\): nếu \(\overrightarrow a .\overrightarrow b = 0\)
+ \(\overrightarrow a \) và \(\overrightarrow b \) cùng phương:
\(\left( {\overrightarrow a ,\overrightarrow b } \right) = {0^o}\) nếu \(\overrightarrow a \) và \(\overrightarrow b \) cùng hướng
\(\left( {\overrightarrow a ,\overrightarrow b } \right) = {180^o}\) nếu \(\overrightarrow a \) và \(\overrightarrow b \) ngược hướng
Nếu không thuộc các trường hợp trên thì ta tính góc dựa vào công thức \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}}\).
HĐ2
Cho hai vectơ cùng phương \(\overrightarrow u = \left( {x;y} \right)\) và \(\overrightarrow v = \left( {kx;ky} \right)\). Hãy kiểm tra công thức \(\overrightarrow u .\overrightarrow v = k\left( {{x^2} + {y^2}} \right)\) theo từng trường hợp sau:
a) \(\overrightarrow u = \overrightarrow 0 \)
b) \(\overrightarrow u \ne \overrightarrow 0 \) và \(k \ge 0\)
c) \(\overrightarrow u \ne \overrightarrow 0 \) và \(k < 0\)
Phương pháp giải:
Tính tích vô hướng bằng công thức: \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
Lời giải chi tiết:
a) Vì \(\overrightarrow u = \overrightarrow 0 \) nên \(\overrightarrow u \) vuông góc với mọi \(\overrightarrow v \).
Như vậy \(\overrightarrow u .\overrightarrow v = 0\)
Mặt khác: \(\overrightarrow u = \overrightarrow 0 \Leftrightarrow x = y = 0\)
\( \Rightarrow k\left( {{x^2} + {y^2}} \right) = 0 = \overrightarrow u .\overrightarrow v \)
b) Vì \(\overrightarrow u \ne \overrightarrow 0 \) và \(k \ge 0\) nên \(\overrightarrow u \) và \(\overrightarrow v \)cùng hướng.
\( \Rightarrow \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = {0^o} \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = 1\)
\(\begin{array}{l} \Rightarrow \overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right| = \sqrt {{x^2} + {y^2}} .\sqrt {{{\left( {kx} \right)}^2} + {{\left( {ky} \right)}^2}} \\ = \sqrt {{x^2} + {y^2}} .\left| k \right|.\sqrt {{x^2} + {y^2}} = k\left( {{x^2} + {y^2}} \right)\end{array}\)
(|k|= k do k > 0)
c) Vì \(\overrightarrow u \ne \overrightarrow 0 \) và \(k < 0\) nên \(\overrightarrow u \) và \(\overrightarrow v \)ngược hướng.
\( \Rightarrow \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = {180^o} \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = - 1\)
\(\begin{array}{l} \Rightarrow \overrightarrow u .\;\overrightarrow v = - \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right| = - \sqrt {{x^2} + {y^2}} .\sqrt {{{\left( {kx} \right)}^2} + {{\left( {ky} \right)}^2}} \\ = - \sqrt {{x^2} + {y^2}} .\left| k \right|.\sqrt {{x^2} + {y^2}} = k\left( {{x^2} + {y^2}} \right).\end{array}\)
HĐ3
Trong mặt phẳng tọa độ Oxy, cho hai vectơ không cùng phương \(\overrightarrow u = \left( {x;y} \right)\) và \(\overrightarrow v = \left( {x';y'} \right)\).
a) Xác định tọa độ của các điểm A và B sao cho \(\overrightarrow {OA} = \overrightarrow u ,\;\overrightarrow {OB} = \overrightarrow v .\)
b) Tính \(A{B^2},O{A^2},O{B^2}\) theo tọa độ của A và B.
c) Tính \(\overrightarrow {OA} .\overrightarrow {OB} \) theo tọa độ của A, B.
Lời giải chi tiết:
a) Vì \(\overrightarrow {OA} = \overrightarrow u = (x;y)\) nên A(x; y).
Tương tự: do \(\overrightarrow {OB} = \overrightarrow v = \left( {x';y'} \right)\) nên B (x’; y’)
b) Ta có: \(\overrightarrow {OA} = (x;y) \Rightarrow O{A^2} = {\left| {\overrightarrow {OA} } \right|^2} = {x^2} + {y^2}.\)
Và \(\overrightarrow {OB} = (x';y') \Rightarrow O{B^2} = {\left| {\overrightarrow {OB} } \right|^2} = x{'^2} + y{'^2}.\)
Lại có: \(\overrightarrow {AB} = \overrightarrow {OB} - \overrightarrow {OA} = \left( {x';y'} \right) - \left( {x;y} \right) = \left( {x' - x;y' - y} \right)\)
\( \Rightarrow A{B^2} = {\left| {\overrightarrow {AB} } \right|^2} = {\left( {x' - x} \right)^2} + {\left( {y' - y} \right)^2}.\)
c) Theo định lí cosin trong tam giác OAB ta có:
\(\cos \widehat O = \frac{{O{A^2} + O{B^2} - A{B^2}}}{{2.OA.OB}}\)
Mà \(\overrightarrow {OA} .\overrightarrow {OB} = \left| {\overrightarrow {OA} } \right|.\left| {\overrightarrow {OB} } \right|.\cos \left( {\overrightarrow {OA} ,\overrightarrow {OB} } \right) = OA.OB.\cos \widehat O\)
\( \Rightarrow \overrightarrow {OA} .\overrightarrow {OB} = OA.OB.\frac{{O{A^2} + O{B^2} - A{B^2}}}{{2.OA.OB}} = \frac{{O{A^2} + O{B^2} - A{B^2}}}{2}\)
\(\begin{array}{l} \Rightarrow \overrightarrow {OA} .\overrightarrow {OB} = \frac{{{x^2} + {y^2} + x{'^2} + y{'^2} - {{\left( {x' - x} \right)}^2} - {{\left( {y' - y} \right)}^2}}}{2}\\ \Leftrightarrow \overrightarrow {OA} .\overrightarrow {OB} = \frac{{ - \left( { - 2x'.x} \right) - \left( { - 2y'.y} \right)}}{2} = x'.x + y'.y\end{array}\)
Luyện tập 3
Tích vô hướng và góc giữa hai vectơ \(\overrightarrow u = \left( {0; - 5} \right),\;\overrightarrow v = \left( {\sqrt 3 ;1} \right)\)
Phương pháp giải:
Cho \(\overrightarrow u = \left( {x;y} \right)\) và \(\overrightarrow v = \left( {x';y'} \right)\), khi đó: \(\overrightarrow u .\overrightarrow v = x.x' + y.y'\)
Lời giải chi tiết:
Ta có: \(\overrightarrow u = \left( {0; - 5} \right),\;\overrightarrow v = \left( {\sqrt 3 ;1} \right)\)
\( \Rightarrow \overrightarrow u .\;\,\overrightarrow v = 0.\sqrt 3 + \left( { - 5} \right).1 = - 5.\)
HĐ4
Cho ba vectơ \(\overrightarrow u = ({x_1};{y_1}),\;\overrightarrow v = ({x_2};{y_2}),\;\overrightarrow w = ({x_3};{y_3}).\)
a) Tính \(\overrightarrow u .\left( {\overrightarrow v + \overrightarrow w } \right),\;\overrightarrow u .\overrightarrow v + \overrightarrow u .\overrightarrow w \) theo tọa độ của các vectơ \(\overrightarrow u ,\overrightarrow v ,\overrightarrow w .\)
b) So sánh \(\overrightarrow u .\left( {\overrightarrow v + \overrightarrow w } \right)\) và \(\;\overrightarrow u .\overrightarrow v + \overrightarrow u .\overrightarrow w \)
c) So sánh \(\;\overrightarrow u .\overrightarrow v \) và \(\overrightarrow v .\overrightarrow u \)
Phương pháp giải:
Cho \(\overrightarrow u = \left( {x;y} \right)\) và \(\overrightarrow v = \left( {x';y'} \right)\), khi đó: \(\overrightarrow u .\overrightarrow v = x.x' + y.y'\)
Lời giải chi tiết:
a) Ta có: \(\overrightarrow u = ({x_1};{y_1}),\;\overrightarrow v = ({x_2};{y_2}),\;\overrightarrow w = ({x_3};{y_3}).\)
\(\begin{array}{l} \Rightarrow \overrightarrow v + \overrightarrow w = ({x_2};{y_2}) + ({x_3};{y_3}) = \left( {{x_2} + {x_3};{y_2} + {y_3}} \right)\\ \Rightarrow \overrightarrow u .\left( {\overrightarrow v + \overrightarrow w } \right) = {x_1}.\left( {{x_2} + {x_3}} \right) + {y_1}.\left( {{y_2} + {y_3}} \right)\end{array}\)
Và: \(\;\overrightarrow u .\overrightarrow v + \overrightarrow u .\overrightarrow w = \left( {{x_1}.{x_2} + {y_1}.{y_2}} \right) + \left( {{x_1}.{x_3} + {y_1}.{y_3}} \right)\)\( = {x_1}.{x_2} + {y_1}.{y_2} + {x_1}.{x_3} + {y_1}.{y_3}.\)
b) Vì \({x_1}.{x_2} + {y_1}.{y_2} + {x_1}.{x_3} + {y_1}.{y_3}\)\( = \left( {{x_1}.{x_2} + {x_1}.{x_3}} \right) + \left( {{y_1}.{y_2} + {y_1}.{y_3}} \right)\)\( = {x_1}.\left( {{x_2} + {x_3}} \right) + {y_1}.\left( {{y_2} + {y_3}} \right)\)
Nên \(\overrightarrow u .\left( {\overrightarrow v + \overrightarrow w } \right) = \;\overrightarrow u .\overrightarrow v + \overrightarrow u .\overrightarrow w \)
c) Ta có: \(\overrightarrow u = ({x_1};{y_1}),\;\overrightarrow v = ({x_2};{y_2})\)
\( \Rightarrow \left\{ \begin{array}{l}\overrightarrow u .\overrightarrow v = {x_1}.{x_2} + {y_1}.{y_2}\\\overrightarrow v .\overrightarrow u = {x_2}.{x_1} + {y_2}.{y_1}\end{array} \right.\)\( \Leftrightarrow \;\overrightarrow u .\overrightarrow v = \overrightarrow v .\overrightarrow u \)
Luyện tập 4
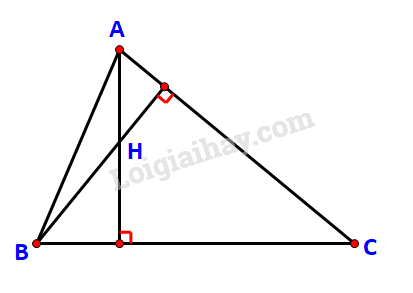
Cho tam giác ABC với A(-1; 2), B(8; -1), C(8; 8). Gọi H là trực tâm của tam giác.
a) Chứng minh rằng \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \) và \(\overrightarrow {BH} .\overrightarrow {CA} = \overrightarrow 0 \)
b) Tìm tọa độ của H.
c) Giải tam giác ABC.
Phương pháp giải:
a) \(\overrightarrow u \bot \overrightarrow v \Leftrightarrow \overrightarrow u .\overrightarrow v = 0\)
b) Lập hệ PT biết \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \) và \(\overrightarrow {BH} .\overrightarrow {CA} = \overrightarrow 0 \).
c) Nếu vectơ \(\overrightarrow {AB} (x;y) \) thì \(\left| {\overrightarrow {AB} } \right| = \sqrt {{x^2} + {y^2}} \)
Lời giải chi tiết:

a) \( AH \bot BC\) và \(BH \bot CA\)
\( \Rightarrow \left( {\overrightarrow {AH} ,\overrightarrow {BC} } \right) = {90^o} \Leftrightarrow \cos \left( {\overrightarrow {AH} ,\overrightarrow {BC} } \right) = 0\) . Do đó \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \)
Tương tự suy ra \(\overrightarrow {BH} .\overrightarrow {CA} = \overrightarrow 0 \).
b) Gọi H có tọa độ (x; y)
\( \Rightarrow \left\{ \begin{array}{l}\overrightarrow {AH} = (x - ( - 1);y - 2) = (x + 1;y - 2)\\\overrightarrow {BH} = (x - 8;y - ( - 1)) = (x - 8;y + 1)\end{array} \right.\)
Ta có: \(\overrightarrow {AH} .\overrightarrow {BC} = \overrightarrow 0 \) và \(\overrightarrow {BC} = (8 - 8;8 - ( - 1)) = (0;9)\)
\((x + 1).0 + (y - 2).9 = 0 \Leftrightarrow 9.(y - 2) = 0 \Leftrightarrow y = 2.\)
Lại có: \(\overrightarrow {BH} .\overrightarrow {CA} = \overrightarrow 0 \) và \(\overrightarrow {CA} = ( - 1 - 8;2 - 8) = ( - 9; - 6)\)
\(\begin{array}{l}(x - 8).( - 9) + (y + 1).( - 6) = 0\\ \Leftrightarrow - 9x + 72 + 3.( - 6) = 0\\ \Leftrightarrow - 9x + 54 = 0\\ \Leftrightarrow x = 6.\end{array}\)
Vậy H có tọa độ (6; 2)
c) Ta có: \(\overrightarrow {AB} = (8 - ( - 1); - 1 - 2) = (9; - 3)\)\( \Rightarrow AB = \left| {\overrightarrow {AB} } \right| = \sqrt {{9^2} + {{( - 3)}^2}} = 3\sqrt {10} \)
Và \(\overrightarrow {BC} = (0;9) \Rightarrow BC = \left| {\overrightarrow {BC} } \right| = \sqrt {{0^2} + {9^2}} = 9\);
\(\overrightarrow {CA} = ( - 9; - 6)\)\( \Rightarrow AC = \left| {\overrightarrow {CA} } \right| = \sqrt {{{( - 9)}^2} + {{( - 6)}^2}} = 3\sqrt {13} .\)
Áp dụng định lí cosin cho tam giác ABC, ta có:
\(\cos \widehat A = \frac{{{b^2} + {c^2} - {a^2}}}{{2bc}} = \frac{{{{\left( {3\sqrt {13} } \right)}^2} + {{\left( {3\sqrt {10} } \right)}^2} - {{\left( 9 \right)}^2}}}{{2.3\sqrt {13} .3\sqrt {10} }} \approx 0,614\)\( \Rightarrow \widehat A \approx 52,{125^o}\)
\(\cos \widehat B = \frac{{{a^2} + {c^2} - {b^2}}}{{2ac}} = \frac{{{{\left( 9 \right)}^2} + {{\left( {3\sqrt {10} } \right)}^2} - {{\left( {3\sqrt {13} } \right)}^2}}}{{2.9.3\sqrt {10} }} = \frac{{\sqrt {10} }}{{10}}\)\( \Rightarrow \widehat B \approx 71,{565^o}\)
\( \Rightarrow \widehat C \approx 56,{31^o}\)
Vậy tam giác ABC có: \(a = 9;b = 3\sqrt {13} ;c = 3\sqrt {10} \); \(\widehat A \approx 52,{125^o};\widehat B \approx 71,{565^o};\widehat C \approx 56,{31^o}.\)
Vận dụng
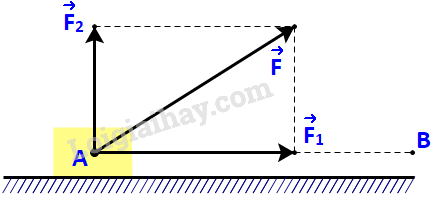
Một lực \(\overrightarrow F \) không đổi tác động vào một vật và điểm đặt của lực chuyển động thẳng từ A đến B. Lực \(\overrightarrow F \) được phân tích thành hai lực thành phần là \(\overrightarrow {{F_1}} \) và \(\overrightarrow {{F_2}} \) \((\overrightarrow F = \overrightarrow {{F_1}} + \overrightarrow {{F_2}} \;).\)
a) Dựa vào tính chất của tích vô hướng, hãy giải thích vì sao công sinh bởi lực \(\overrightarrow F \) (đã được đề cập ở trên) bằng tổng của các công sinh bởi các lực \(\overrightarrow {{F_1}} \) và \(\overrightarrow {{F_2}} \).
b) Giả sử các lực thành phần \(\overrightarrow {{F_1}} \), \(\overrightarrow {{F_2}} \)tương ứng cùng phương, vuông góc với phương chuyển động của vật. Hãy tìm mối quan hệ giữa các công sinh bởi lực \(\overrightarrow F \) và lực \(\overrightarrow {{F_1}} \).
Phương pháp giải:
Khi lực \(\overrightarrow F \) không đổi tác dụng lên một vật và điểm đặt chuyển dời một đoạn s theo hướng hợp với hướng của lực góc α thì công sinh bởi lực đó là: \(A = F.{\rm{ }}s.\cos \alpha \)
Lời giải chi tiết:
a)

Gọi \(A,{A_1},{A_2}\) lần lượt là công sinh bởi lực \(\overrightarrow F \), \(\overrightarrow {{F_1}} \) và \(\overrightarrow {{F_2}} \).
Ta cần chứng minh: \(A = {A_1} + {A_2}\)
Xét lực \(\overrightarrow F \), công sinh bởi lực \(\overrightarrow F \) là: \(A = \left| {\overrightarrow F } \right|.{\rm{ AB}}.\cos \left( {\overrightarrow F ,\overrightarrow {AB} } \right) = \overrightarrow F .\overrightarrow {AB} \)
Tương tự, ta có: \({A_1} = \overrightarrow {{F_1}} .\overrightarrow {AB} \), \({A_2} = \overrightarrow {{F_2}} .\overrightarrow {AB} \)
Áp dụng tính chất của tích vô hướng ta có:
\({A_1} + {A_2} = \overrightarrow {{F_1}} .\overrightarrow {AB} + \overrightarrow {{F_2}} .\overrightarrow {AB} = \left( {\overrightarrow {{F_1}} + \overrightarrow {{F_2}} } \right).\overrightarrow {AB} = \overrightarrow F .\overrightarrow {AB} = A\)
b)
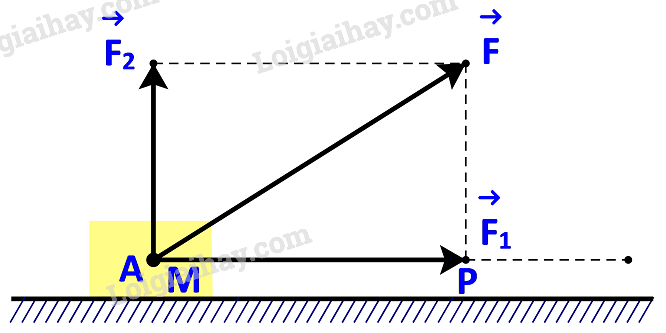
Vì \(\overrightarrow {{F_2}} \)tương ứng vuông góc với phương chuyển động nên \(\overrightarrow {{F_2}} \bot \overrightarrow {AB} \)
Do đó: công sinh bởi lực \(\overrightarrow {{F_2}} \) là: \({A_2} = \overrightarrow {{F_2}} .\overrightarrow {AB} = 0\)
Mà \(A = {A_1} + {A_2}\)
\( \Rightarrow A = {A_1}\)
Vậy công sinh bởi lực \(\overrightarrow F \) bằng công sinh bởi lực \(\overrightarrow {{F_1}} \).
Câu hỏi 1
Khi nào thì tích vô hướng của hai vectơ \(\overrightarrow u ,\;\overrightarrow v \) là một số dương? Là một số âm?
Phương pháp giải:
+) Tích vô hướng của hai vectơ \(\overrightarrow u ,\;\overrightarrow v \): \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
Nhận xét: \(\overrightarrow u .\;\overrightarrow v \) cùng dấu với \(\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
Lời giải chi tiết:
Dễ thấy: \(\overrightarrow u .\;\overrightarrow v \) cùng dấu với \(\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\) (do \(\left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right| > 0\)). Do đó:
+) \(\overrightarrow u .\;\overrightarrow v \;\; > 0\) \( \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) > 0\) hay \({0^o} \le \left( {\overrightarrow u ,\;\overrightarrow v } \right) < {90^o}\)
+) \(\overrightarrow u .\;\overrightarrow v \;\; < 0\) \( \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\;\; < 0\) hay \({90^o} < \left( {\overrightarrow u ,\;\overrightarrow v } \right) \le {180^o}\)
Vậy \(\overrightarrow u .\;\overrightarrow v \;\; > 0\) nếu \({0^o} \le \left( {\overrightarrow u ,\;\overrightarrow v } \right) < {90^o}\) và \(\overrightarrow u .\;\overrightarrow v \;\; < 0\) nếu \({90^o} < \left( {\overrightarrow u ,\;\overrightarrow v } \right) \le {180^o}.\)
Câu hỏi 2
Khi nào thì \({\left( {\overrightarrow u .\;\overrightarrow v } \right)^2} = {\left( {\overrightarrow u } \right)^2}.{\left( {\overrightarrow v } \right)^2}\)?
Phương pháp giải:
+) \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
+) \({\overrightarrow u ^2} = {\left| {\overrightarrow u } \right|^2}\) với mọi vectơ \(\overrightarrow u \)
Lời giải chi tiết:
\({\left( {\overrightarrow u .\;\overrightarrow v } \right)^2} = {\left( {\overrightarrow u } \right)^2}.{\left( {\overrightarrow v } \right)^2}\)\( \Leftrightarrow {\left[ {\left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)} \right]^2} = {\left| {\overrightarrow u } \right|^2}.{\left| {\overrightarrow v } \right|^2}\)
\(\begin{array}{l} \Leftrightarrow {\left[ {\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)} \right]^2} = 1\\ \Leftrightarrow \left[ \begin{array}{l}\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = 1\\\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = - 1\end{array} \right.\end{array}\)
\( \Leftrightarrow \left[ \begin{array}{l}\;\left( {\overrightarrow u ,\;\overrightarrow v } \right) = {0^o}\\\left( {\overrightarrow u ,\;\overrightarrow v } \right) = {180^o}\end{array} \right.\)
Hay hai vectơ \(\overrightarrow u ,\;\overrightarrow v \) cùng phương.
Vậy hai vectơ \(\overrightarrow u ,\;\overrightarrow v \) cùng phương thì \({\left( {\overrightarrow u .\;\overrightarrow v } \right)^2} = {\left( {\overrightarrow u } \right)^2}.{\left( {\overrightarrow v } \right)^2}\)
Luyện tập 2
Cho tam giác AB C có BC = a, CA = b, AB = c. Hãy tính \(\overrightarrow {AB} .\overrightarrow {AC} \) theo a,b,c.
Phương pháp giải:
+) Tích vô hướng: \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
Lời giải chi tiết:
Ta có: \(\overrightarrow {AB} .\overrightarrow {AC} = \left| {\overrightarrow {AB} } \right|.\left| {\overrightarrow {AC} } \right|.\cos \left( {\overrightarrow {AB} ,\overrightarrow {AC} } \right)\)
Mà \(\left( {\overrightarrow {AB} ,\overrightarrow {AC} } \right) = \widehat {BAC}\)\( \Rightarrow \cos \left( {\overrightarrow {AB} ,\overrightarrow {AC} } \right) = \cos \widehat {BAC}\)
Lại có: \(\cos \widehat {BAC} = \frac{{{b^2} + {c^2} - {a^2}}}{{2bc}}\)(suy ra từ định lí cosin)
\(\begin{array}{l} \Rightarrow \overrightarrow {AB} .\overrightarrow {AC} = AB.AC.\frac{{{b^2} + {c^2} - {a^2}}}{{2bc}}\\ \Leftrightarrow \overrightarrow {AB} .\overrightarrow {AC} = c.b.\frac{{{b^2} + {c^2} - {a^2}}}{{2bc}}\\ \Leftrightarrow \overrightarrow {AB} .\overrightarrow {AC} = \frac{{{b^2} + {c^2} - {a^2}}}{2}\end{array}\)
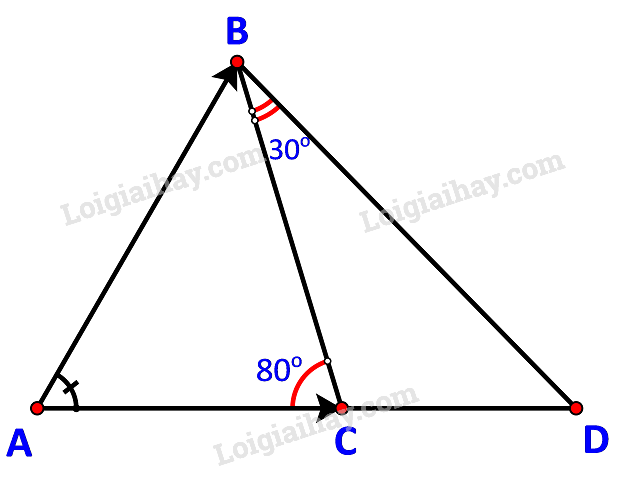
HĐ1
Trong hình 4.39, số đo góc BAC cũng được gọi là số đo góc giữa hai vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {AC} \). Hãy tìm số đo các góc giữa \(\overrightarrow {BC} \) và \(\overrightarrow {BD} \), \(\overrightarrow {DA} \) và \(\overrightarrow {DB} \).
Lời giải chi tiết:
Góc giữa hai vectơ \(\overrightarrow {BC} \) và \(\overrightarrow {BD} \) là góc CBD và số đo \(\widehat {CBD} = {30^o}\).
Góc giữa hai vectơ \(\overrightarrow {DA} \) và \(\overrightarrow {DB} \) là góc ADB.
Ta có: \(\widehat {ACB} = \widehat {CBD} + \widehat {CDB}\) (tính chất góc ngoài)
\(\begin{array}{l} \Leftrightarrow \widehat {CDB} = {80^o} - {30^o} = {50^o}\\ \Leftrightarrow \widehat {ADB} = {50^o}\end{array}\)
Vậy số đo góc giữa hai vectơ \(\overrightarrow {BC} \) và \(\overrightarrow {BD} \), \(\overrightarrow {DA} \) và \(\overrightarrow {DB} \) lần lượt là \({30^o},{50^o}\)
Câu hỏi
Khi nào thì góc giữa hai vectơ bằng \({0^o}\), bằng \({180^o}?\)
Phương pháp giải:
Cách xác định góc giữa hai vecto \(\overrightarrow u ,\overrightarrow v \)
Lấy điểm A bất kì vẽ \(\overrightarrow {AB} = \overrightarrow u ,\overrightarrow {AC} = \overrightarrow v \), khi đó \((\vec u,\vec v) = (\overrightarrow {AB} ,\overrightarrow {AC} ) = \widehat {BAC}\)
Lời giải chi tiết:
Góc giữa hai vectơ bằng \({0^o}\) nếu chúng cùng hướng
Góc giữa hai vectơ bằng \({180^o}\) nếu chúng ngược hướng.
Luyện tập 1
Cho tam giác đều ABC. Tính \(\left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right)\).
Phương pháp giải:
Lấy D sao cho: \(\overrightarrow {AD} = \overrightarrow {BC} \).
Khi đó: \(\left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right) = \left( {\overrightarrow {AB} ,\overrightarrow {AD} } \right) = \widehat {BAD}\)
Lời giải chi tiết:
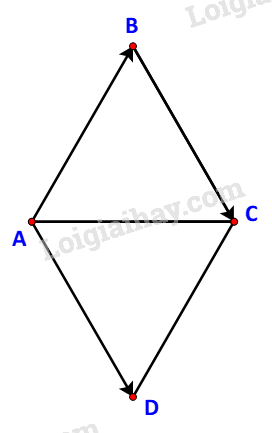
Lấy điểm D sao cho: \(\overrightarrow {AD} = \overrightarrow {BC} \)
Khi đó ta có: \(\left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right) = \left( {\overrightarrow {AB} ,\overrightarrow {AD} } \right) = \widehat {BAD}\)
Dễ thấy ABCD là hình bình hành (hơn nữa còn là hình thoi) nên \(\widehat {BAD} = {180^o} - \widehat {ABC} = {120^o}\)
Vậy số đo góc \(\left( {\overrightarrow {AB} ,\overrightarrow {BC} } \right)\) là \({120^o}\).
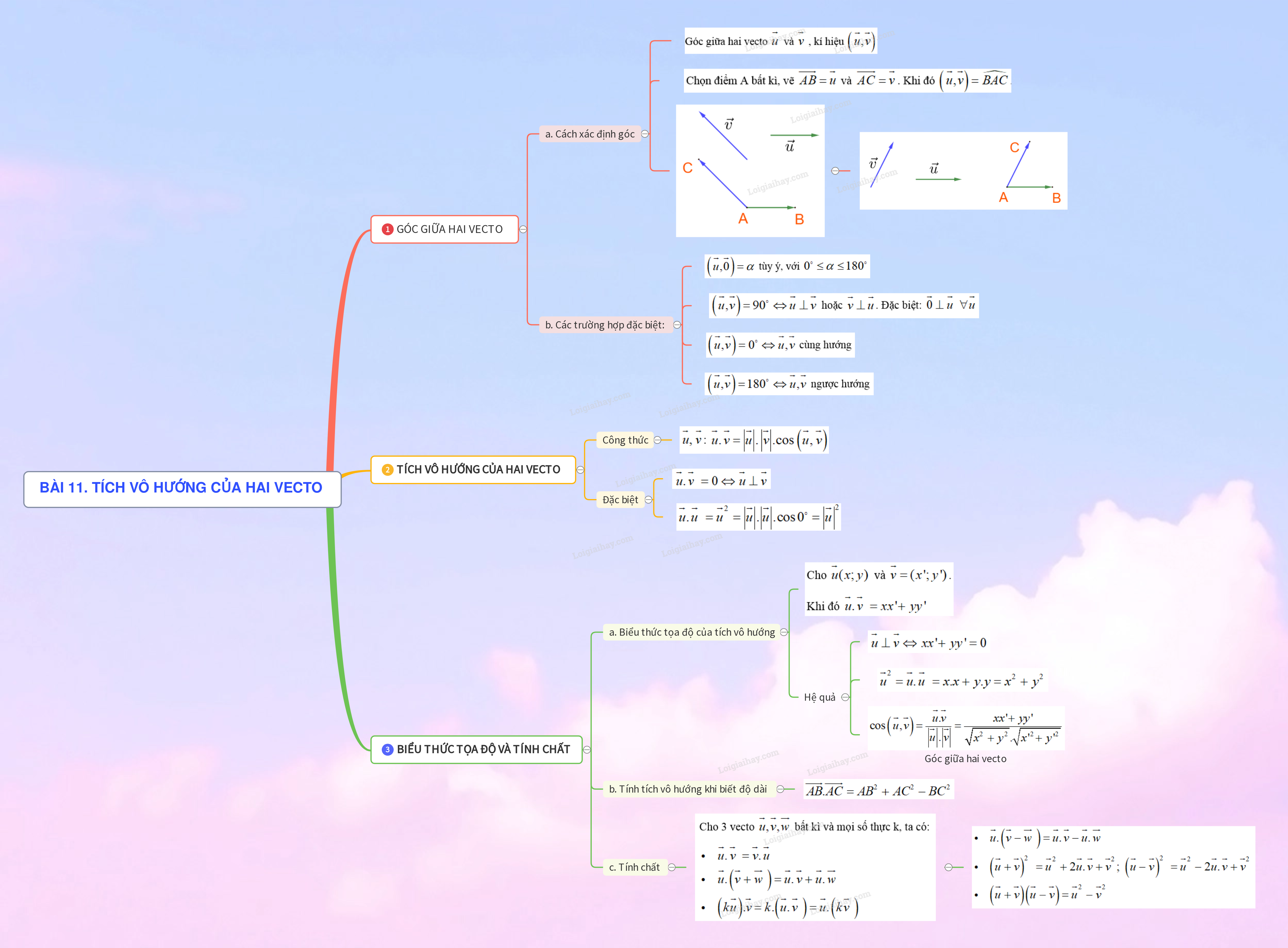
1. GÓC GIỮA HAI VECTO
Cho hai vecto \(\overrightarrow u \) và \(\overrightarrow v \) khác \(\overrightarrow 0 \). Góc giữa hai vecto \(\overrightarrow u \) và \(\overrightarrow v \) , kí hiệu \(\left( {\;\overrightarrow u ,\overrightarrow v } \right)\)
a) Cách xác định góc: Chọn điểm A bất kì, vẽ \(\overrightarrow {AB} = \overrightarrow u \) và \(\overrightarrow {AC} = \overrightarrow v \). Khi đó \(\left( {\;\overrightarrow u ,\overrightarrow v } \right) = \widehat {BAC}\).

b) Các trường hợp đặc biệt:
+) \(\left( {\;\overrightarrow u ,\overrightarrow 0 } \right) = \alpha \) tùy ý, với \({0^ \circ } \le \alpha \le {180^ \circ }\)
+) \(\left( {\;\overrightarrow u ,\overrightarrow v } \right) = {90^ \circ } \Leftrightarrow \overrightarrow u \bot \overrightarrow v \) hoặc \(\overrightarrow v \bot \overrightarrow u \). Đặc biệt: \(\overrightarrow 0 \bot \overrightarrow u \;\;\forall \overrightarrow u \;\)
+) \(\left( {\;\overrightarrow u ,\overrightarrow v } \right) = {0^ \circ } \Leftrightarrow \overrightarrow u ,\overrightarrow v \) cùng hướng
+) \(\left( {\;\overrightarrow u ,\overrightarrow v } \right) = {180^ \circ } \Leftrightarrow \overrightarrow u ,\overrightarrow v \) ngược hướng
2. TÍCH VÔ HƯỚNG CỦA HAI VECTO
+) Tích vô hướng của hai vecto \(\overrightarrow u ,\;\overrightarrow v \): \(\overrightarrow u .\;\overrightarrow v = \left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right|.\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\)
+) \(\overrightarrow u .\;\overrightarrow v \;\; = 0 \Leftrightarrow \overrightarrow u \bot \;\overrightarrow v \;\;\)
+) \(\overrightarrow u .\;\overrightarrow u \;\; = {\overrightarrow u ^2} = \left| {\overrightarrow u } \right|.\left| {\overrightarrow u } \right|.\cos {0^ \circ } = {\left| {\overrightarrow u } \right|^2}\)
3. BIỂU THỨC TỌA ĐỘ VÀ TÍNH CHẤT CỦA TÍCH VÔ HƯỚNG
a) Biểu thức tọa độ của tích vô hướng
Cho \(\overrightarrow u (x;y)\) và \(\overrightarrow v = (x';y')\).
Khi đó \(\overrightarrow u .\;\overrightarrow v \;\; = xx' + yy'\)
Hệ quả:
+) \(\overrightarrow u \bot \;\overrightarrow v \; \Leftrightarrow xx' + yy' = 0\)
+) \({\overrightarrow u ^2} = \overrightarrow u .\;\overrightarrow u \;\; = x.x + y.y = {x^2} + {y^2}\)
+) Tìm góc giữa hai vecto: \(\cos \left( {\;\overrightarrow u ,\overrightarrow v } \right) = \frac{{\;\overrightarrow u .\overrightarrow v }}{{\;\left| {\overrightarrow u } \right|.\left| {\overrightarrow v } \right|}} = \frac{{xx' + yy'}}{{\sqrt {{x^2} + {y^2}} .\sqrt {x{'^2} + y{'^2}} }}\)
b) Công thức tính tích vô hướng khi biết độ dài:
Theo định lí cosin: \(\cos \widehat {BAC} = \frac{{A{B^2} + A{C^2} - B{C^2}}}{{2.AB.AC}}\)
\( \Rightarrow \overrightarrow {AB} .\overrightarrow {AC} = AB.AC.\cos \widehat {BAC} = A{B^2} + A{C^2} - B{C^2}\)
c) Tính chất
Cho 3 vecto \(\overrightarrow u ,\overrightarrow v ,\overrightarrow w \) bất kì và mọi số thực k, ta có:
\(\begin{array}{l}\overrightarrow u .\;\overrightarrow v \;\; = \overrightarrow v .\;\overrightarrow u \;\\\overrightarrow u .\;\left( {\overrightarrow v + \overrightarrow w \;} \right)\; = \overrightarrow u .\;\overrightarrow v \; + \overrightarrow u .\;\overrightarrow w \;\\\left( {k\overrightarrow u } \right).\overrightarrow v = k.\left( {\overrightarrow u .\;\overrightarrow v \;} \right) = \overrightarrow u .\;\left( {k\overrightarrow v \;} \right)\end{array}\)
Hệ quả
\(\begin{array}{l}\overrightarrow u .\;\left( {\overrightarrow v - \overrightarrow w \;} \right)\; = \overrightarrow u .\;\overrightarrow v \; - \overrightarrow u .\;\overrightarrow w \\{\left( {\overrightarrow u + \overrightarrow v } \right)^2}\;\; = {\overrightarrow u ^2} + 2\overrightarrow u .\;\overrightarrow v \; + \;{\overrightarrow v ^2};\;\;{\left( {\overrightarrow u - \overrightarrow v } \right)^2}\;\; = {\overrightarrow u ^2} - 2\overrightarrow u .\;\overrightarrow v \; + \;{\overrightarrow v ^2}\\\left( {\overrightarrow u + \overrightarrow v } \right)\left( {\overrightarrow u - \overrightarrow v } \right) = {\overrightarrow u ^2} - {\overrightarrow v ^2}\end{array}\)